Interest rate risk is risk to the earnings or market value of a portfolio due to uncertain future interest rates. Discussions of interest rate risk can be confusing because there are two fundamentally different ways of approaching the topic. People who are accustomed to one often have difficulty grasping the other. The two perspectives are:

• a book value perspective, which perceives risk in terms of its effect on accounting earnings, and
• a market value perspective—sometimes called an economic perspective—which perceives risk in terms of its effect on the market value of a portfolio.

The first perspective is typical in banking, insurance and corporate treasuries, where book value accounting prevails. The latter is typical in a trading or investment management context.

Interest rate risks can be categorized in different ways, and there is usually some overlap between categories. One approach—that is well suited for a book-value perspective—is to break interest rate risk into three components:

• term structure risk,
• basis risk,
• options risk.

Term structure risk (also called yield curve risk or repricing risk) is risk due to changes in the fixed income term structure. It arises if interest rates are fixed on liabilities for periods that differ from those on offsetting assets. One reason may be maturity mismatches. Suppose an insurance company is earning 6% on an asset supporting a liability on which it is paying 4%. The asset matures in two years while the liability matures in ten. In two years, the firm will have to reinvest the proceeds from the asset. If interest rates fall, it could end up reinvesting at 3%. For the remaining eight years, it would earn 3% on the new asset while continuing to pay 4% on the original liability. Term structure risk also occurs with floating rate assets or liabilities. If fixed rate assets are financed with floating rate liabilities, the rate payable on the liabilities may rise while the rate earned on the assets remains constant.

In general, any occasion on which interest rates are to be reset—either due to maturities or floating rate resets—is called a repricing. The date on which it occurs is called the repricing date. It is this terminology that motivates the alternative name "repricing risk" for tem structure risk.

If a portfolio has assets repricing earlier than liabilities, it is said to be asset sensitive. This is because near term changes in earnings are going to be driven by interest rate resets on those assets. Similarly, if liabilities reprice earlier, earnings are more exposed to interest rate resets on those liability, and the portfolio is called liability sensitive.

For example, a bank that is supporting fixed rate liabilities with floating rate assets is asset sensitive. Earnings risk is posed by the floating rate on the assets. This example is only meaningful from a book value standpoint—which focuses on earnings risk. From a market risk standpoint, the floating rate assets pose little risk—floaters have stable market values. It is the long-dated liabilities that pose market risk. Their market values fluctuate with changes in long-term interest rates. From the economic perspective, it would be reasonable to call the bank "liability sensitive!" Of course, that is not how the terminology is used. However, our example highlights how fundamentally different the book-value and market-value perspectives are.

It should be emphasized that this discussion uses the terms "asset" and "liability" loosely, and not in any strict accounting sense. We include among assets and liabilities both derivatives and other off-balance sheet instruments that may behave like assets or liabilities. A pay-fixed interest rate swap might be considered a combination of a floating rate asset with a fixed rate liability. On a stand-alone basis, it poses considerable term structure risk.

Basis risk is risk due to possible changes in spreads. In fixed income markets, basis risk arises form changes in the relationship between interest rates for different market sectors. If a bank makes loans at prime while financing those loans at Libor, it is exposed to the risk that the spread between prime and Libor may narrow. If a portfolio holds junk bonds hedged with short Treasury futures, it is exposed to basis risk due to possible changes in the yield spread of junk bonds over Treasuries.  Basis risk is another name for spread risk.

As with term structure risk, book-value and market-value perspectives differ with respect to basis risk. As always, the book value perspective focuses on risk to earnings. If the spread between interest earned on assets and interest paid on liabilities narrows, those earnings will suffer. The economic perspective considers the risk to the portfolio's market value. If a spread narrows or widens, the market values of assets and liabilities may be affected differently—and the net market value of the overall portfolio could suffer.

Options risk, as a component of interest rate risk, is risk due to fixed income options—options that have fixed income instruments or interest rates as underliers. Options may be stand-alone, such as caps or swaptions. They may also be embedded, as with the call feature of callable bonds or the prepayment of mortgage-baked securities (MBS). In some respects, options risk is just another component of term structure risk. This argument needs to be explored differently for the book value and market value perspectives.

From the book value perspective, the distinction between term structure and options risk has historical roots. Payoffs of options depends upon changes in interest rates, which would seem to make options one more source of term structure risk. However, by shorting embedded options, a depository institution can enhance short-term earnings at the expense of long-term earnings. This is what happened during the 1980s, when the MBS market was just emerging. Dealers found US thrifts and other depository institutions to be eager buyers of MBSs. Because of their short embedded prepayment options, the MBSs offered very high yields—and those high yields flowed immediately to earnings. Because MBS pricing was far from transparent, dealers could charge exorbitant prices for the MBS—they priced them to have yields much higher than Treasury notes, but not high enough to fully compensate for the short options. From an economic standpoint, thrifts incurred a loss every time they purchased an MBS, but the thrifts didn't see that. Perceiving the world from a purely book-value/earnings perspective, all they saw was an immediate jump in earnings. Only later, when interest rates dropped and prepayments on the MBS surged, did the thrifts realize their mistake. Loses were staggering and were a primary contributor to the ensuing crisis in the US thrift industry.

Part of the thrifts' problem was due to being cheated by the dealers who sold them the MBS at inflated prices. That is a risk distinct from interest rate risk. It is as old as Wall Street—caveat emptor. However, another significant issue was the emerging problem that derivatives and new structures with embedded options made it possible to do an "end-run" around traditional book value accounting. Increasingly, earnings could be manipulated for the short-term, with consequences pushed into the future. Traditional techniques of asset-liability management—which focused on term structure and basis risk—were ill equipped to address this emerging risk. Hence, the new risk was given a name—options risk—and managers came under pressure to supplement old tools with new ones that could assess this new risk.

The economic perspective on options risk is very different. From that standpoint, options pose immediate risk in the form of changes in their market value. While shorting embedded options can generate income that immediately flows to earnings, it does nothing for market value—the option premiums are offset by the negative market value of the newly shorted options. If the options are shorted at fair prices, the two cancel—and there is no immediate market value impact.

Market risk of fixed income options arises primarily from two sources:

• changes in underlying interest rates, and
• changes in applicable implied volatilities.

The first of these, from a market value standpoint, is no different from term structure risk—the portfolio's value rises or falls with interest rates in a fairly predictable manner. The latter isn't a form of interest rate risk—it is implied volatility risk. Accordingly, from an economic perspective, it is more reasonable to identify just two components of interest rate risk:

• term structure risk, and
• basis risk.

where a term structure includes a component of what we previously called options risk, and the balance of options risk is a new, non-interest rate risk:

• volatility risk.

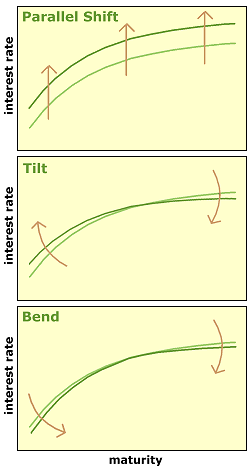
There are many techniques for assessing interest rate risk. Some focus on the earnings impact of interest rate risk. Others focus on the market value impact. Accordingly, the choice of tools will be motivated by your perspective.

Investors with a book value perspective tend to address interest rate risk with the tools of asset-liability management—cash matching, gap analysis, earnings simulation, earnings at risk and duration. Those with an economic perspective use some of these—especially gap analysis and duration—but they also use tools that focus on economic value—delta, PV01 and value-at-risk.

Tools such as earnings simulation and earnings-at-risk quantify risk in terms of its earnings impact, so they are only applicable from a book-value perspective. Tools like PV01 and value-at-risk quantify risk in terms of market value impact, so they are only applicable from a market-value perspective. Gap analysis and duration are interesting because they can be used with either perspective. Let's look at why.

Gap analysis doesn't consider the consequences of the risk it assesses, so it doesn't lock the user into one perspective or the other. It simply identifies interest rate gaps. The book-value and market-value perspectives may see differing implications in those gaps, but they both see risk. Accordingly, gap analysis is useful for both.

Duration is different. Rather than avoid describing the consequences of the risks it identifies, it offers two alternative interpretations of those risks. Based on the Macaulay formula, duration is the average weighted maturity of a portfolio. From the book-value perspective, a portfolio that has positive duration is liability sensitive. One that has negative duration is asset sensitive. From the economic perspective, duration describes the sensitivity of the portfolio's market value to parallel shifts in the spot curve. Accordingly, the single notion of duration is perceived in fundamentally different ways.

As mentioned earlier, there is no particular need from an economic perspective to consider a separate options risk. Standard tools, including delta, vega, PV01 and value-at-risk—if correctly implemented—easily capture the risks of options. From a book value perspective, traditional tools, including cash matching and gap analysis, simply cannot incorporate options. This necessitated a search for new tools. One was earnings simulation. If implemented to assess risk over a long-enough horizon—in the past, it often wasn't— it can easily incorporate the effects of options over time. Another is option-adjusted duration. Abandoning the somewhat limited Macaulay formula, investors would use option pricing models to accurately calculate duration as a factor sensitivity. To clarify terminology, from the book value perspective, duration is Macaulay duration and option-adjusted duration is what, from an economic perspective, is called duration. Yes, interest rate risk can be confusing. Blame it on the two competing perspectives.

Spread risk is risk (usually market risk or earnings risk) due to exposure to some spread. It often arises with a long-short position or with derivatives. A synonym for spread risk is basis risk.

Suppose a bank lends at prime and finances itself at Libor. It faces spread risk due to the possibility that the prime-Libor spread might narrow. A bond trader might hedge a long position in corporate bonds by shorting Treasury bonds. The hedge eliminates exposure to changes in Treasury yields, but the trader remains exposed to changes in the spread between corporate and Treasury yields. He too is taking spread risk. See the article Interest Rate Risk for more on basis risk in fixed income markets.

If futures are used to hedge a long or short position in an underlier, residual risk will remain due to the spread between the futures price and the underliers spot price. That spread is called the futures' basis.

Gap analysis is a technique of asset-liability management that can be used to assess interest rate risk or liquidity risk. Implementations for those two applications differ in minor ways, so people distinguish between interest rate gaps and liquidity gaps. This article discusses both.

Gap analysis was widely adopted by financial institutions during the 1980s. When used to manage interest rate risk, it was used in tandem with duration analysis. Both techniques have their own strengths and weaknesses.

Duration is appealing because it summarizes, with a single number, exposure to parallel shifts in the term structure of interest rates. It does not address exposure to other term structure movements, such as tilts or bends. Gap analysis is more cumbersome and less widely applicable, but it assesses exposure to a greater variety of term structure movements.

Changes in the Term Structure of Interest Rates Exhibit 1

The term structure of interest rates can move in many ways. Duration analysis addresses exposure to parallel shifts only. Gap analysis can warn of exposure to more complex movements, including tilts and bends.

Let's start our discussion with cash flow matching (or simply cash matching). This is an effective, but largely impractical means of eliminating interest rate risk. If a portfolio has a positive fixed cash flow at some time t, its market value will increase or decrease inversely with changes in the spot interest rate for maturity t. If the portfolio has a negative fixed cash flow at time t, it's market value will increase or decrease in tandem with changes in that spot rate. Stated simply, interest rate risk arises from either positive or negative net future cash flows. The concept of cash matching is to eliminate interest rate risk by eliminating all net future cash flows. A portfolio is cash matched if

• every future cash inflow is balanced with an offsetting cash outflow on the same date, and
• every future cash outflow is balanced with an offsetting cash inflow on the same date.

The net cash flow for every date in the future is then 0. Obviously, this is an ideal that we usually don't want to achieve, but it is a theoretically useful concept. In its most basic form, gap analysis assesses how close a portfolio is to being cash matched. Here is how it works.

Start by considering a portfolio with only fixed cash flows—that is, the timing and amount of all cash flows is known. The portfolio contains no floaters, no options and no bonds with embedded options. Soon we will loosen the restriction against floaters, but let's keep it for now. Gap analysis doesn't consider credit risk, so assume all cash flows will occur.

Gap analysis comprises aggregating cash flows into maturity buckets and checking if cash flows in each bucket net to 0. Different bucketing schemes might be used. As a simple example, consider a portfolio whose cash flows all mature in less than three years. We aggregate maturities into five buckets:

• 0 - 3 months
• 3 - 6 months
• 6 - 12 months
• 12 - 24 months
• 24 - 36 months

An interest rate gap is simply a positive or negative net cash flow for one of the buckets. Exhibit 2 illustrates a gap analysis using our buckets and some hypothetical cash flows.

Example: Gap Analysis Exhibit 2

Bucketed cash flows in USD millions. A gap is any net cash flow for a bucket, so there is a USD 100MM gap for the 3 - 6 month bucket. There is a negative gap of USD 30MM for the 12 - 24 month bucket.

Note that this portfolio is exposed to tilts in the term structure of interest rates. If rates for the 3 - 6 month bucket rise and rates for the 12 - 24 month bucket decline, the portfolio will incur a mark-to-market loss on both gaps. This exposure would not be identified by duration. If you calculate the Macaulay duration of the portfolio, it is about 0.

Now let's add floating rate instruments to the portfolio. These generally are not bucketed according to their maturity but according to their next reset date. Consider a USD 100MM floating rate note (FRN) that pays 3-month Libor flat. Its last reset was a month ago at 2.8%. It will pay USD 0.7MM in two months, and then the rate will be reset again.

From a market value standpoint, the FRN is equivalent to a fixed cash payment of USD 100.7MM to be received in two months. Accordingly, that is how we bucket it—we bucket the entire FRN as a single USD 100.7MM cash flow in the 0 - 3 month bucket.

Because of how floaters are treated, buckets are often called repricing buckets as opposed to maturity buckets—instruments are bucketed according to their next repricing date as opposed to their maturity date. We are moving away from cash matching and towards repricing date matching. From this standpoint, interest rate gaps are sometimes called repricing gaps.

So far, we have discussed the use of gap analysis for assessing interest rate risk. It can also be used to assess liquidity risk. Cash flows are bucketed as above. The only difference is that cash flows from floaters are bucketed according to their maturity. The actual values of floating rate cash flows will not be known, but estimated values may be used. The idea of liquidity gap analysis is to anticipate periods when a portfolio will have large cash out-flows. Such buckets are called liquidity gaps.

A shortcoming of gap analysis—both interest rate and liquidity gap analysis—is the fact that it does not identify mismatches within buckets. An even more significant shortcoming is the fact that it cannot handle options in a meaningful way. In today's markets, options proliferate. Fixed income portfolios routinely hold caps, floors, swaptions, mortgage-backed securities, callable bonds, etc. Options have cash flows whose magnitudes—and sometimes timing—is highly uncertain. Those uncertain cash flows cannot be bucketed. For this reason, gap analysis has largely fallen out of use. Today, gap analysis is most useful as a theoretical tool for communicating issues related to interest rate and liquidity risk.

(Source: RiskGlossary.com)

[Home] [Virtual Library] [Articles] [GRG Services] [Topics] [Internal Auditing] [Risk Management] [Asset Management] [IAS-IFRS] [Risk Jobs] [News] [Softwares] [Books] [Sponsors] [Links] [Contact Us] [Feedback] [Blog]

Copyright ©2005-2009 Global Risk Guard. All rights reserved. | Legal |